Lift Force and Friction Force
Streamlined features are indeed a very confounded and troublesome part of liquid elements, yet on the off chance that we dismiss every one of the troublesome subtleties, the basics are additionally straightforward for standard individuals. How does a plane fly?
The flow of air on an airplane wing. The wing receives a lift force upwards and experiences a frictional force backwards.
Stream of air on a plane wing. The wing gets a lift power upwards and encounters a frictional power in reverse. A plane wing has such a shape that wind currents quicker along the wing at the top than at the base. As indicated by Bernoulli's regulation, air streaming at a low speed has a higher tension. This implies that the pneumatic stress at the lower part of the wing is higher than at the top. The outcome is a power upward upwards: the lift power. Furthermore, the wing likewise gets a frictional power to the back. On the off chance that the lift power on the wings is equivalent to the power of gravity on the airplane, the airplane can keep on flying evenly. As it turns out, a thought with Bernoulli's regulation isn't the entire story. The wing gets power from the air upwards. Then, at that point, as per Newton's third regulation (activity = - response), there should be a similarly incredible descending power of the airplane on the air. The wing along these lines pushes air downwards and consequently gets a power itself upwards. Behind the wing, the wind streams diagonally downwards. The extent of the lift power is given by the recipe:
In this recipe, An is the wing region, v is the speed of the airplane comparative with the air, ρ is the thickness of the air and the dimensionless consistent cL is known as the lift coefficient. The recipe is basic, however, all the streamlined features issues are concealed in the cL. The specific worth of cL relies upon many subtleties of the wind current around the wings and fuselage. Subtleties that the experts in airplane development are worried about. Notwithstanding Lift Force, the wing likewise gets a rubbing power that can be determined by the accompanying recipe:
The importance of the letters is equivalent to the equation for the lift power. A compact disc is the drag coefficient (the D is from the English word 'drag'). With air stream tests and at times roughly by hypothetical estimation, cL and cD are still up in the air. The coefficients firmly rely upon the purported 'approach', a sort of approach: the point between the harmony of the wing and the heading of stream of the air.
The 'angle of attack', is the angle between the inflowing air and the 'chord' (chord).
The 'approach', is the point between the coming air and the 'harmony' (harmony). In the figure inverse, cL and cD are given as an element of the approach. Somewhere in the range of 0o and 15o cL increments significantly, while CD remaining parts about something very similar. However, above 15o the lift coefficient drops pointedly, while the drag increments tremendously. This is called slow down (slow down in English). The wind stream can never again follow the wing totally and vortices are made at the top. Slow down is what is happening for the airplane: the wing loses a lot of lift power and gets a great deal of obstruction. Assuming that the plane flies low, it could crash on slow down. Values of cL and cD as a component of the approach
As indicated by the diagram, around 10o is a protected approach: there is adequate lift power and the risk of slowing down isn't entirely ideal. Stream around the wing without slowing down Stream around the wing with cover. Vertebrae are made over the wings and the lift power is lost. The proportion cL/cD decides the streamlined quality. It gives generally how much lift the wing has for one newton of drag. For a herring gull, that quality variable is around 12, for a Boeing 747 16, and for a gooney bird 20. A lightweight flyer or a super-ultralight could get 35. Increasing a herring gull If a bird/plane/fly bicycle flies evenly, the lifting power should be equivalent to gravity, so † Utilizing this equation we can really look at what occurs assuming we scale a herring gull with a mass of 1 kg to a monster of 100 kg. In the equation for the lift power, just v and An are variable. So on the off chance that m becomes multiple times as huge, the item v2 * An absolute necessity additionally be multiple times as huge. In the event that the mass becomes multiple times as huge, the volume will likewise increment to multiple times, in light of the fact that the thickness of the bird won't actually change. That implies that length, width, and tallness each become 3,100 = 4.6 times bigger. The wing region A then, at that point, becomes 4.62 = 21.5 times as enormous. Also, v2 should then become 100/21.5 = 4.6 times as enormous, so v becomes = 2.15 times as huge. On the off chance that we duplicate the mass by multiple times, becomes wingspan 4.6 times bigger the wing region is 21.5 times as enormous. Furthermore, the speed should be 2.15 times quicker. We apply this to the herring gull. The Herring Gull has a mass of 1 kg and a wingspan of 1.4 m, a wing area of 0.2 m2, and a cruising rate of 12 m/s. A gull multiple times as weighty has a wingspan of 4.6 * 1.4 = 6.5 meters and a wing




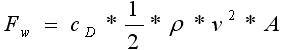






No comments:
Post a Comment